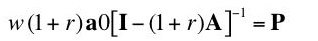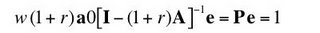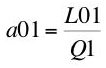I Couldn't Make This Up

Right on cue, Greg Mankiw exemplifies the intellectual impoverishment of mainstream teaching in economics.

I hadn't previously read Gabriel Mihalache's blog. I'm inclined to be more tolerant of this nonsense, given this acknowledgement. (Is it clear that the equations in this post are clickable?)

Based on the comments on this post, I may have more readers than the amount of comments suggests.

Foley On Incoherence Of Marginalist Project

Here are two long quotations from Duncan K. Foley, "Value, Distribution and Capital: A Review Essay", Review of Political Economy, V. 13, N. 3, 2001.

"The strongest and most important point that has come out of the classical critique of marginalism and neoclassical economics is its refutation of the capital scarcity theory of the rate of profit.

The notion that the profit rate can be coherently viewed as being determined by a 'marginal product of capital' given by technology and input availabilities is one of the more confused and ideologically muddied chapters in the history of economics. A cost-minimizing firm facing a wage, cost of capital, and prices of capital goods determined by markets will adapt its relative use of labor and capital to those prices. The value of capital goods is a rational and appropriate measure of capital input to the firm when the prices of capital goods are determined by market forces outside the firm's control. When a cost-minimizing firm faces a range of technical methods of production that approximate a smooth continuum of capital-labor ratios, cost-minimization entails setting the marginal value product of each input equal to its price. (There is, of course, vigorous debate in all schools of economic thought over how well the assumptions of a cost-minimizing firm facing market prices for inputs fits the behavior of real capitalist firms.) In this scenario, however, it is the wage and the cost of capital that determine the marginal value products of labor and capital, not the other way around.

The vision of the marginalists and their neoclassical followers was that this uncontroversial theorem of cost minimization could somehow be transformed into a theory of the wage and profit rate, and hence into a theory of distribution. Their hopes of accomplishing this transformation stemmed from their anthropomorphic vision of the economy and its markets as analogous to the allocation of scarce resources by a single decision maker with a well-defined objective function, who, at least under the assumptions of concavity of the objective function and convexity of resource constraints, can impute shadow prices (Lagrange multipliers) to the resources. This program, despite firing the imaginations of many talented economists, has never managed to disentangle the problems of aggregation of disparate preferences, treatment of time and information, definition of resource limitations, and dynamic stability of market clearing that are inherent in carrying it out to arrive at the robust, unified, and transparent account of distribution it sought. Economics owes a particular debt of gratitude (which it shows precious few signs of recognizing, to tell the truth) to Sraffa and his followers for their dogged insistence on bringing to light the ramifying incoherence of this marginalist project...

...The Cambridge capital debate centered initially on one aspect of this tangle of confusions, the neoclassical hope that the value of capital goods would somehow behave like a single scarce input in equilibrium. The interchanges of this debate, including an important paper by Garegnani (1970), showed unambiguously that the neoclassical construction would work in general only under assumptions on production so stringent as to amount to the assumption of a single capital good. Other work related to the Cambridge debate, for example that of Luigi Pasinetti (1974) and Stephen Marglin (1984), also underlined the other side of Sraffa's critique, the necessity of taking some distributional variable, either the wage or profit rate, as the boundary condition in production models, rather than stocks of individual capital goods. This path clarifies the real relationship between input prices and marginal productivities (if indeed they exist), which is that input prices determine marginal productivities through the cost-minimizing choice of technology.

Neoclassical economists have a hard time keeping their minds clear on this point. They are distracted by the fact that it is possible to embed a Sraffian production system in a general equilibrium model with given stocks of inputs and calculate equilibrium prices and rates of return without any reference to the value of capital goods. They read this model as supporting the scarcity theory of profit rates, although it fact it only reproduces its own assumption of the full employment of all inputs except those that have zero prices in equilibrium. The equilibrium allocation can equally well be viewed as one in which given input prices determine cost-minimizing choices of technique that happen to be compatible with arbitrarily given supplies of inputs. In the absence of a compelling dynamic theory that shows how the market might find these prices, which neoclassical theory has pretty well given up hope for, Sraffa's critique carries the day.

It would have been a good thing if the Cambridge capital controversy had managed to drive a stake through the heart of the scarcity theory of the rate of profit, but it hasn't. I think this has been because the classical critique tells economists what they shouldn't do (assume full employment and market clearing in order to determine prices) but doesn't tell them very clearly what they should do as an alternative, either at the purely theoretical level or in econometric studies."

Those who have followed this series of posts with attention will understand, to some extent, Foley's statement that "input prices determine marginal productivities through the cost-minimizing choice of technology."

Marginal Productivity Not A Theory Of Distribution (Part 4)

6.0 Conclusions
Let me recap. In the first part, I laid out a model of the economy. In the second part, I derived marginal productivity conditions and noted equations for equilibrium prices. In the third part, I outlined how to construct the factor price frontier, and asserted that that analysis is equivalent to marginal productivity analysis when they both are applicable.

Marginal productivity is not a theory of income distribution. I consider it an analysis, like the construction of the factor price frontier, of the choice of technique.

Consider two nested models of production. In the first, the coefficients of production are fixed. Equilibrium conditions in the production model would consist of the system of equations arising from the condition that no pure economic profit exists. These equilibrium conditions and the choice of the numeraire determine, with one degree of freedom, the wage, the interest rate, and prices. No marginal productivity conditions exist, for interior solutions, in this model.

In the more general model, one allows coefficient of production to vary, instead of taking them as given parameters. Marginal productivity equilibrium conditions arise for each coefficient of production. The model still has a single degree of freedom.

If one took the wage as given from outside, the model shows how firms choose processes in which the present value of the marginal product of labor is equal to the wage. The wage is not determined by the value of the marginal product of labor. Rather, it is more appropriate to say the value of the marginal product of labor is determined by the wage.

Samuelson, however, when calculating the marginal product of labor, noted that the interest rate could be taken as given instead. The factor price frontier shows possible distributions of income, given the possible coefficients of production. But marginal productivity cannot determine a specific location on the frontier. By taking an important distributive variable, the interest rate, as given, an economist, in theory, can determine the location on that frontier and the value of the marginal product of labor. (Notice that in case of reswitching, one cannot even map from the chosen technique, out of the set of all possible techniques, to the wage and the value of the marginal product of labor.)

Interestingly enough, Walras understood that marginal productivity is not a theory of income distribution:

"[The theory of marginal productivity] introduces into the problem of production a system of equations...in which the number of equations is equal to the number of coefficients of production and in which these coefficients are represented as unknowns...[The theory] makes possible a definitive criticism and refutation of the English theory of rent, by showing that the consideration of marginal productivity is relevant to the determination of coefficients of production, but is not relevant to the determination of the price of services." (Leon Walras, Elements of Pure Economics, Appendix III, (Translated by William Jaffe), 1954.)

Occasionally, you may see somebody saying that changes in technology or relative physical productivity are what drives changes in income distribution, at least in theory:

"To a good neoclassical economist, the statement that the relative price of a factor of production--like the labor of the elite top 1% of America's wage and salary distribution--has risen is the same thing as the statement that the relative productivity of that factor of production has risen." -- Brad DeLong

Does DeLong get neoclassical economics correct?

For now, that's all.

Marginal Productivity Not A Theory Of Distribution (Part 3)

5.0 The "Factor Price Frontier"
This post builds on an analysis of marginal productivity in an economic model.

One can rewrite Equation 17 as Equation 20:

(20)

Or:

(21)

The matrix inverse exists for all r between zero and some maximum, inclusive, for each technique if both goods are basic and the economy is viable.

Let e be a column vector denoting the numeraire. Post-multiplying, one obtains:

(22)

Hence, the wage-rate of interest curve for a given technique is:

(23)

This is a downward sloping curve in w-r space, and it cuts both axis.

One can plot the wage-rate of interest curve for each technique. A technique is a set of coefficients of production, a0 and A. The coefficients of production a01, a11, a21, for the wheat industry lie on the unit-isoquant production function for wheat. Likewise, the coefficients of production a02, a12, a22, for the corn industry lie on the unit-isoquant production function for corn. Given a common numeraire used in plotting each curve, these curves fall in the same space, as in this example.

Consider the frontier formed by the outer envelope of all these curves. That is, for a given interest rate, the wage on the factor price frontier is the maximum wage from out of the wages on all the wage-interest rate curves at that rate of interest. Similarly, for a given wage, the factor price frontier shows the maximum rate of interest. And the coefficients of production used in constructing wage-rate of interest curves on the frontier for, say, a given rate of interest can be chosen by competitive profit-maximizing (or cost-minimizing) firms paying the given rate of interest and the equilibrium wage thereby determined.

The coefficients of production found in this analysis of the factor price frontier are the same as those that arise in the marginal productivity analysis. When the production functions are well-behaved and continuously differentiable, no point on the frontier is a switch point, and the optimizing coefficients of production vary continuously with the interest rate. Since the two analyses, of the factor price frontier and of marginal productivity, are equivalent, the wage is equal to the present value of the marginal product of labor for every point on the frontier.

The analyses of the factor price frontier, however, can be used to determine the cost-minimizing coefficients of production when the industry-specific production functions are not smooth. That is, the factor price frontier can be constructed when the set of possible coefficients of production is discrete. In this sense, the analysis of the factor price frontier, inasmuch as it applies to both continuously differentiable production functions and a discrete model of production, is more general than an analysis confined to cases where derivatives exist. (This is not a radical conclusion; post (Second World) war mainstream economists do not confine themselves to calculus-based results; they have long used topological arguments.)

Marginal Productivity Not A Theory Of Distribution (Part 2)

3.0 Marginal Conditions Arising From Profit Maximization

Previously, I outlined a model on an economy in which wheat and corn are produced in yearly production cycles. If the profit-maximizing competitive firm produces wheat, it must solve the following mathematical programming problem:

Given w, P(1), P(2)
Choose a01, a11, a21 to
To Maximize
(6)
Such that

The solution gives the following marginal productivity equations:

(7)

(8)

(9)

In competitive equilibrium, the price of every input is equal to the value of the marginal product for each product.

Pure economic profit must be nonnegative, for if it were negative the firm would have chosen not to produce at all. It works out that pure economic profit cannot be positive either. Equation 10 states that pure economic profit is zero in the wheat-producing industry:

(10)

An analogous set of three marginal productivity equations and a zero economic profit condition arises from analyzing corn-producing firms.

4.0 Interest Rates In Steady States
Consider the following definitions:

(11)

(12)

These are known as the own rates of interest of wheat and corn, respectively.

In a long run equilibrium, the quantities of wheat and corn inputs are not given. Assume they have been adjusted such that relative spot prices of corn, wheat, and labor remain unchanged from year to year. Almost all economists up until the late 1920s, as far as I am aware, thought of this constancy of relative prices, or an equivalent condition, as a defining property of long run equilibrium.

(It was about then that short-run notions of temporary and intertemporal equilibrium were introduced, and the economists' conceptions became more complex. Mistakes were made by the early neoclassicals. Walras thought he could take endowments of produced goods as given, but still consider an equilibrium with steady-state prices. Others mistakenly wanted to take the quantity of "capital" as given, but have its form, in terms of composition of wheat and corn, be endogenously determined.)

Certainly, we need to introduce some condition relating industries. Anyways, If you think about the condition of stationary spot prices, you will see that if no pure economic profits are possible, relative forward and relative spot prices must be equal:

(13)

Otherwise, an agent could buy or sell wheat or corn in the forward market for a year and make a pure economic profit on the spot market at the end of the year.

Therefore:

(14)

Or:

(15)

where r is the common own rate of interest for all commodities. It is not a parameter of some unobservable utility function, although it may become equal to some such parameter in equilibrium in special cases. Such a special case could be created by closing this model with utility-maximization by a infinitely-lived representative agent.

The condition of unchanging relative spot prices allows us to remove P(2) from our equations. We have:

(16)

The condition of no economic profit gives the following system of two equations:

(17)

where I have dropped the time index on the price vector as being no longer needed. The marginal productivity equations become:

(18)

(19)

So we have a system of eight Equations - the two price equations (17), and the six marginal productivity equations (18) and (19). There are ten choice variables to be determined by the model:

• The wage w
• The common own rate of interest r
• Two prices, P = (p1, p2)
• Six production coefficients a0 and A

I assume it's obvious that the above model generalizes to an n good model. There are two degrees of freedom. One is closed by selecting the numeraire.

There is no equation equating r to some mystical, mythical, marginal product of capital.

In the next part, I outline the construction of the so-called factor price frontier.

Marginal Productivity Not A Theory Of Distribution (Part 1)

1.0 Introduction
What is held constant when defining the marginal product of labor? A correct answer, in the context of a neoclassical long period theory, is the interest rate. This is part of the answer Paul Samuelson gave to Joan Robinson in the early 1960s when she visited MIT. My exposition of this answer is indebted to an explanation of Frank Hahn's.

2.0 The Model Economy
Consider an economy that produces wheat and corn in yearly production cycles. At the start of the year, a firm producing wheat buys inputs of labor, corn, and wheat. The production process takes a year. The firm's output of wheat is available at the end of the year. Likewise, a firm buying corn purchases inputs of labor, corn, and wheat at the start of the year and has outputs of corn at the end of the year.

In neoclassical theory, goods are differentiated by their availability at different times. So there will be five prices to consider for a yearly production cycle:

• w, the wage, which I arbitrarily assume here is paid at the beginning of the year.
• P(1) = (p11, p21), the row vector of spot prices of wheat and corn for immediate delivery at the beginning of the year.
• P(2) = (p12, p22), the vector of forward prices of wheat and corn for delivery at the end of the year. For example, p12 is the price that must be paid at the start of the year for a contract in which the buyer will receive a bushel of wheat at the end of the year.

Now consider a competitive firm producing wheat. Assume the firm faces a constant returns to scale production function:

(1)

where Q1 is the output of bushels wheat, L01 is inputs of labor, X11 is inputs of wheat, and X21 is inputs of corn. Since I assume Constant Returns to Scale, I can separate questions of scale and relative proportions:

(2)

where

(3)

(4)

(5)

I make the usual assumption that diminishing marginal returns exist for each input in both production functions.

In the next part, I derive the marginal productivity equations for a competitive wheat-producing firms.

Some of Samuelson on the Subject of Sraffa

Samuelson in 1966:

”Such an unconventional behavior of the capital/output ratio is seen to be definitely possible. It can perhaps be understood in terms of so-called Wicksell and other effects. But no explanation is needed for that which is definitely possible: it demonstrates itself. Moveover, this phenomenon can be called ‘perverse’ only in the sense that the conventional parables did not prepare us for it….

…Nevertheless we must accept nature as she is. In a general blueprint technology model of Joan Robinson and M.I.T. type, it is quite possible to encounter switch points … in which lower profit rates are associated with lower steady-state capital/output ratios…

…Pasinetti, Morishima, Bruno-Burmeister-Sheshinski, Garegnani merit our gratitude for demonstrating that reswitching is a logical possibility in any technology, indecomposable or decomposable.”

In 1971:

”In this age of Leontief and Sraffa there is no excuse for mystery or partisan polemics in dealing with the purely logical aspects of the problem.”

In 1975:

”…one who believes technology to be more like my 1966 reswitching example than like its orthodox contrast, will have a more sanguine view about how successful militant power by organized labor can be in causing egalitarian shifts in the distribution of income away from property even in the long run.”

In 1976:

”Those who have followed the literature of the past 20 years know that these many properties of the Clarkian paradigm do not all hold generally. In every Cambridge, and wherever informed economic theory is taught, there is no 1976 disagreement on this this.”

In 1987:

”Oscar Lange once wrote, sardonically but seriously, that Ludwig von Mises, the enemy of socialism, deserved a statue in the socialist Hall of Fame for compelling Abba Lerner and Lange (to say nothing of earlier Pareto, Barone and Fred Taylor) to work out how socialists might devise efficient decentralizing-pricing planning algorithms. One can insist, seriously, that a neoclassical Hall of Fame deserves Sraffa’s statue…

…Did any scholar have so great an impact on economic science as Piero Sraffa did in so few writings? One doubts it. And there cannot be many scholars in any field whose greatest works were published in their second half century of life.

Piero Sraffa was much respected and much loved. With each passing year, economists perceive new grounds for admiring his genius.”

In 2001:

”The Nobel Prize of Piero Sraffa and Joan Robinson that Stockholm never awarded might have pleased at least one of them. Its citation would have included: ‘Their investigations uncovered a fatal normative flaw in Bohm-Bawerkian and modern mainstream capital theory’…

…As my exposition will reveal, the fatal flaw in question can exist even when a scalar ‘leets’ is the sole producible input; it can exist even when precise neoclassical marginal products do exist and do serve to pin down unequivocally the distribution of incomes between propertyless workers and affluent capitalists. The statues of Piero and Joan belong in the pantheon of neoclassicism itself…

…this defense does not validate an inverse [interest rate, consumption per person-hour] tradeoff… Adam Smith’s Invisible Hand does ensure Equation (5) above but cares nought for Equation (6). This is why books entitled Economics in One Lesson must evoke from us the advice: ‘Go back for the second lesson’.”

But doubtless tyro economists are taught today all about the contributions of an economist who had an age co-named after him. Apparently these contributions lead to the acceptance that class struggle can affect distribution under neoclassical theory. To the thesis that mainstream capital theory is “fatally flawed”. To an understanding of what capital-reversing and reswitching of techniques are. And to a realization that these phenomenon are compatible with the assumptions of mainstream economics.

• Samuelson, P. A. (1966). “A Summing Up”, Quarterly Journal of Economics, V. 80, N. 4 (Nov): 568-583
• Samuelson, P. A. (1971). “Understanding the Marxian Notion of Exploitation: A Summary of the So-Called Transformation Problem Between Marxian Values and Competitive Prices”, Journal of Economic Literature, V. 9, N. 2 (Jun.): 399-431”
• Samuelson, P. A. (1975). “Steady-State and Transient Relations: A Reply on Reswitching”, Quarterly Journal of Economics, V. 89, N. 1 (Feb.): 40-47
• Samuelson, P. A. (1976). “Interest Rate Determinations and Oversimplifying Parables: A Summing Up” in Essays in Modern Capital Theory (edited by M. Brown, K. Sato, and P. Zambreka), Amsterdam: North-Holland
• Samuelson, P. A. (1987). “Sraffian Economics” in The New Palgrave: A Dictionary of Economics (edited by J. Eatwell, M. Milgate, and P. Newman),London: Macmillan
• Samuelson, P. A. (2001). “A Modern Post-Mortem on Bohm’s Capital Theory: Its Vital Normative Flaw Shared By Pre-Sraffian Mainstream Capital Theory”, Journal of the History of Economic Thought, V. 23, N. 3: 301-317

To Show The Fly The Way Out Of The Flybottle

"There is a sense in which [William] James was not a philosopher at all. He once said to me: 'What a curse philosophy would be if we couldn't forget all about it!' In other words, philosophy was not to him what it has been to so many, a consolation and sanctuary in a life which would have been unsatisfying without it. It would be incongruous, therefore, to expect of him that he would build a philosophy like an edifice to go and live in for good. Philosophy to him was rather like a maze in which he happened to find himself wandering, and what he was looking for was the way out." --George Santayana (quoted in Cornel West, The American Evasion of Philosophy: A Genealogy of Pragmatism, 1989)

Value in Ricardo

In his Principles, Ricardo distinguishes between "market prices" and "natural prices", a distinction he takes from Adam Smith and Smith's predecessors. Market prices are the prices that prevail at any given time on the market. According to classical economists, they include transient and random variations. Natural prices act as centers of gravitational attraction for market prices. At any time, market prices are tending towards natural prices, in some sense. But does the mature Ricardo distinguish, as Karl Marx later does, between natural prices and value, where value is the quantity of labor embodied in a commodity when outputs are at levels consistent with natural prices? (Smith refers to this level as the level of "effectual demand".)

This is an area where Ricardo is notoriously difficult to understand. Consider, however, this passage from towards the end of the first chapter of the third edition of Ricardo's Principles, that is, after Ricardo has explained that natural prices will generally not be proportional to labor values:

"It is not by the absolute quantity of produce obtained by either class, that we can correctly judge of the rate of profit, rent, and wages, but by the quantity of labour required to obtain that produce. By improvements in machinery and agriculture, the whole produce may be doubled; but if wages, rent, and profit be also doubled, these three will bear the same proportions to one another as before, and neither could be said to have relatively varied. But if wages partook not of the whole of this increase; if they, instead of being doubled, were only increased one-half; if rent, instead of being doubled, were only increased three-fourths, and the remaining increase went to profit, it would, I apprehend, be correct for me to say, that rent and wages had fallen while profits had risen; for if we had an invariable standard by which to measure the value of this produce, we should find that a less value had fallen to the class of labourers and landlords, and a greater to the class of capitalists, than had been given before. We might find, for example, that though the absolute quantity of commodities had been doubled, they were the produce of precisely the former quantity of labour. Of every hundred hats, coats, and quarters of corn produced, if

The labourers had before . . . . 25

The landlords . . . . . . . . 25

And the capitalists . . . . . . 50

...And if, after these commodities were double the quantity, of
every 100

The labourers had only . . . . . 22

The landlords . . . . . . . . 22

And the capitalists . . . . . . 56

In that case I should say, that wages and rent had fallen and profits risen; though, in consequence of the abundance of commodities, the quantity paid to the labourer and landlord would have increased in the proportion of 25 to 44. Wages are to be estimated by their real value, viz. by the quantity of labour and capital employed in producing them, and not by their nominal value either in coats, hats, money, or corn. Under the circumstances I have just supposed, commodities would have fallen to half their former value, and if money had not varied, to half their former price also. If then in this medium, which had not varied in value, the wages of the labourer should be found to have fallen, it will not the less be a real fall, because they might furnish him with a greater quantity of cheap commodities than his former wages." (Ricardo 1821)

Furthermore, Sraffa had the good fortune to discover a paper that Ricardo had been working on in the last month of his life, the manuscript "Absolute Value and Exchangable Value". Sraffa has this to say about that manuscript:

"Yet this paper has importance since it develops an idea which existed previously in Ricardo's writings only in occasional hints and allusions: namely, the notion of a real or absolute value underlying and contrasted with exchangeable or relative value." (Sraffa 1951)

Thus, there is textual evidence that, in some places, Ricardo distinguished between price, whether market or natural, and value.

Others have noticed:

"The Marxian reading of Ricardo has fared somewhat better. Marx claimed that Ricardo's treatment of value in the third edition of the Principles was marred by serious confusion: in some places Ricardo used 'value', 'absolute value' or 'real value' to denote 'necessary labour time'; but in others 'value' signified (relative) cost of production. Furthermore, it was Marx's judgement that Ricardo occasionally and falsely identified his 'value' concepts. However, in the opinion of later authorities, including Marshall, Stigler and Steedman, this reading is wholly untenable: Ricardo's 'value' concept was unambiguously one of cost of production.

It is certainly true, and acknowledged by all, that Ricardo had a 'value' concept in the sense of (relative) cost of production. But to claim that this was his only 'value' concept has been to perpetuate a one-sided distortion. Even before his embrace of the labour theory, Ricardo had used 'value' in a manner suggesting an 'absolute' entity associated with the facility or difficulty of producing an individual commodity. Once he made the labour theory his own, that association was with the quantity of labour expended in production, at various times denoted by such terms as 'natural value', 'real value', 'positive value' and even, at the expense of great confusion, 'value' itself. This 'labour quantity' species of 'value' both existed in Ricardo's schema and came to be invested with unique significance, especially in the later writings. Perhaps it is appropriate to remind ourselves that soon after the publication of the third edition of the Principles, on which Marx was commenting, Ricard had written to Trower: 'In speaking of exchange value you have not any idea of real value in your mind, I invariably have.' Much needlessly spilled ink might have been saved if Ricardo had made a similar announcement in his Principles.

To an extent, therefore, Marx's interpretation is superior to his critics: Ricardo sometimes did use 'value' in a manner associated with quantity of labour expenditure (as previous commentors, including Trower, the author of the Observations, Samuel Bailey and, in his Unsettled Questions, John Stuart Mill, had observed.)" (Terry Peach, Interpreting Ricardo, Cambridge University Press, 1993.)

Why I Care About Ito Calculus And How I'll Find Out More

Consider the indexed collection of random variables {X(t) | t a non-negative real}. This is a stochastic process in continuous time. A realization of it is just a time series. It makes sense to me to differentiate or integrate such a realization with respect to time. (As I remember it, though, a realization of a Wiener process is (almost always?) continuous and non-differentiable.) But what it would mean to talk about the derivative or integral of the stochastic process itself? As I understand it, this, along with Stochastic Differential Equations, is the subject of Ito or stochastic calculus.

Recently, physicists have been writing on economics. Generally, econophysicists distrust neoclassical economics and think they can do it better. Joseph L. McCauley's Dynamics of Markets: Econophysics and Finance (Cambridge University Press, 2004) is a recent textbook on econophysics. I find it difficult to read precisely where it draws on Ito calculus.

Luckily I have a resource to help me acquire some mathematical background for McCauley's text. Cosma Shalizi explains the fundamentals of Ito Calculus in Lectures 18-20 of his notes for Advanced Probability II or Almost None of Stochastic Processes.

Textbooks For Teaching Non-Neoclassical Economics

Does an ideological or normative bias exist in mainstream teaching or practice? I think Gunnar Myrdal's The Political Element in the Development of Economic Theory is still worth reading on this topic. Much good stuff has been written on this topic since, too.

In my "Creating Two-Good Reswitching Examples", I list some textbooks for teaching the Cambridge Capital Controversy:

• S. Ahmad (1991). Capital in Economic Theory: Neo-classical, Cambridge, and Chaos, Edward Elgar
• C. Bidard (2004). Prices, Reproduction, Scarcity, Cambridge University Press
• E. Burmeister (1980). Capital Theory and Dynamics, Cambridge University Press
• R. M. Goodwin (1970). Elementary Economics from the Higher Standpoint, Cambridge University Press
• S. Keen (2001). Debunking Economics: The Naked Emperor of the Social Sciences Pluto Press
• H. D. Kurz and N. Salvadori (1995). Theory of Production: A Long-Period Analysis, Cambridge University Press
• L. L. Pasinetti (1977). Lectures on the Theory of Production, Columbia University Press
• J. Robinson and J. Eatwell (1973). Introduction to Modern Economics, McGraw-Hill
• V. Walsh and H. Gram (1980). Classical and Neoclassical Theory of General Equilibrium: Historical Origins and Mathematical Structure, Oxford University Press.
• J. E. Woods (1990). The Production of Commodities: An Introduction to Sraffa, Humanities Press International

These textbooks exhibit a range of rigor, level of mathematics, and assumptions about background. Burmeister's sympathies are clearly with the neoclassicals.

The above list reflects my focus on the CCC. But other focii are possible. Apparently David Krep's A Course in Microeconomic Theory is an introductory graduate text organized by game theory. In his competition, game theory is confined to selected chapters, instead of being an organizing principle.

I have seen references to some considerably less mainstream textbooks. Robin Hahnel's The ABC's of Political Economy: A Modern Approach and Hugh Stretton's Economics: A New Introduction are two I have seen some say nice things about.

There are more textbooks for Post Keynesians. A Marxist would have another list of textbooks. So a supply has been available for economists to teach something different. I suggest if the demand had been seen, even more textbooks would be forthcoming; one can find parts of ones put up here and there on the Web. David Colander is worth reading on the question of why mainstream economists do not alter their teaching, but I've already gone on long enough.

Wikipedian Edit War Over Labor Theory Of Value

This last week has seen an edit war over the Wikipedia Entry On The Labor Theory Of Value (LTV). The warriors are one "Cplot" and "Economizer". I helped write an earlier entry. It was not me, however, who introduced the link to my LTV FAQ. The ostensive issue in this argument is how the first paragraph of the entry should define the LTV.

I don't have a decided opinion on either side in this controversy. Economizer does cite the first sentence in my FAQ. On the other hand, Economizer cites only published literature almost half a century old. I'm grateful to have Donald Gordon's 1959 AER article, "What Was the Labor Theory of Value?" brought to my attention. Cplot is correct that this article could be cited on either side of the issue. It draws on what was recent work by Stigler at the time. Sraffa only appears in a footnote as an editor of Ricardo's collected works.

Cplot is correct in noting that some use the word value to mean the labor embodied in a commodity, with no claim that this quantity is proportional to prices. And one is incorrect to hold both of the following simultaneously:

• The LTV is the theory that prices tend to be proportional to quantities of embodied labor
• Marx and the major classical economists believed in the LTV

Cplot might acknowledge, though, that some thought the LTV was a useful simplification. I can see the point, if you want to argue about how central the LTV is to Marx, not to define the theory as a person of straw that Marx explicitly repudiated.

I think the point of the LTV is not to explain prices. It can be used to emphasize:

• The division of labor, not only within a factory, but among all industries or sectors of an economy
• Some of the conditions for smooth reproduction of an economy
• The claim that the source of the returns to capital and land is value added by labor not paid out to workers.

The technical name for the latter claim, that workers are not fully renumerated for their value-added, is exploitation. Marx seemed to be proud of his introduction of the distinction between "labor" and "labor power" in articulating this theory.

The Wikipedia entry on LTV illustrates an issue with Wikipedia. I have seen how this entry is always being changed, sometimes drastically. It will never approach a steady state.

Update (18 August): For general, mostly negative, comments on Wikipedia, listen to Jason Scott's talk, "The Great Failure of Wikipedia".

Towards A Critique Of Austrian Business Cycle Theory

I suppose I ought to point out this paper, which I updated as of today. I think I still have a ways to go.

Stiglitz: Competitive Economies Almost Never Efficient

From Steve Keen's debunking economics page, I find that Stanley Alcorn and Ben Solarz report on "The Autistic Economist" in the Yale Economic Review. A couple of quotes:

"The First Fundamental Theorem of Welfare Economics [asserts] that every competitive equilibrium is efficient. Based on the work in information economics for which he won the Nobel Prize, Stiglitz finds that this most basic claim is not robust to the removal of the assumption that information is perfect... 'Quite contrary to that theorem', Stiglitz concluded, 'competitive economies are almost never efficient.'"

They also quote Stiglitz as saying that "[Economics as taught] in America's graduate schools... bears testimony to a triumph of idology over science." According to the authors:

"Economics courses at the undergraduate level typically place little-to-no emphasis on learning the tools of economic science, instead focusing on teaching algebraic simplifications of actual economic work, and then assigning problem sets in which students plug in values for the different variables. It is good practice, perhaps, for a few specific mathematical techniques, namely constrained maximization, but it is hardly a training in how to think creatively about dealing with the economy. The bedrock of economics as it is taught is not the subject matter - the economy - or even the approach - the neoclassical school of thought - but ideology, as Stiglitz said. The repetition of simplified and vulgarized economic conclusions is the main task of introductory, intermediate, and even some advanced economics courses, and little else sticks with the students."

I haven't read much of Stiglitz on information asymmetries. I do have this article handy:

• David M. G. Newbery and Joseph E. Stigliz (1982). "The Choice of Techniques and the Optimality of Market Equilibrium with Rational Expectations", Journal of Political Economy, V. 90, N. 2 (Apr.): 223-246

Technical Inquiry

I know Russell Standish has responded to a comments on an article he co-wrote with Steve Keen. And I have had some comments on even older posts. But how would you, dear reader, know this?

I believe changes to my blog are being published to a RSS feed. But how can I easily create some sort of default button to put on the right to let readers push to obtain the RSS feed?

And I have seen some blogs list recent comments on the right. How can I do this? Is there some HTML that I can include in my template?

Thanks in advance.

Cookbooks For Workshops Of The Future

I previously mentioned some programs for post-capitalist societies that I think worth exploring. I think I should add John Roemer's A Future for Socialism to my to-read-someday list (Cosma Shalizi gives it a review).

(I draw heavily on Roemer's Analytical Foundations of Marxian Economic Theory in my 2005 Manchester School paper. But that book has little to say about what comes after capitalism. Bertram Schefold reviews Roemer's Analytical Foundations in the September 1983 issue of the Journal of Economic Literature. Duncan Foley reviews Roemer's Future in the June 1996 issue of the JEL.)

The most convincing argument against socialist central planning, in principle, comes from Enrico Barone's "The Ministry of Production in the Collectivist State" (Il Giornale degli Economisti, 1908). Barone argues that all the categories of capitalism must reappear under socialism:

"From what we have seen and demonstrated hitherto, it is obvious how fantastic those doctrines are which imagine that production in the collectivist regime would be ordered in a manner substantially different from those of 'anarchist' production."

"If the Ministry of Production proposes to obtain the collective maximum - which it obviously must, whatever law of distribution may be adopted - all the economic categories of the old regime must reappear, though maybe with other names: prices, salaries, interest, rent, profit, saving, etc. Not only that; but always provided that it wishes to obtain that maximum with the services of which the individuals and the community dispose, the same two fundamental conditions which characterize free competition reappear, and the maximum is more nearly obtained the more perfectly they are realized. We refer, of course, to the conditions of minimum cost of production and the equalization of price to cost of production." -- E. Barone

Ludwig Von Mises' best contribution to economics was to popularize and promote Barone's argument. Barone, however, does not have a propagandizing organization to promote him. So one cannot find a current willing first hand account of being initiated into an intellectual school with Barone as a member.

Amusing Myself By Critiquing Austrians

Occassionally, for amusement I point out errors in posts on forums in which some of the more dogmatic adherents of Austrian economics hang out. Since I wrote the first draft of the Wikipedia entry on Austrian economics, it should be clear that I find something worthwhile in the writings of some Austrian economists. (I also started the Wikipedia entry on Joseph Schumpeter.) But so many of the adherents of Austrianism you can meet on the Internet only produce “irritable mental gestures which seek to resemble ideas”, as Lionel Trilling said about conservatism.

Anyways, you can see some contributions of mine in the comments in the following, if anybody cares:

• On the falsity of the labour theory of value
• On the Wages Fund theory and on Henry Hazlitt
• Earlier on Hazlitt
• A while ago on Linear Programming and economic calculation

One of my favorite polemical thrusts by Piero Sraffa is this: "Dr. Hayek, who extols the imaginary achievements of the 'subjective method' in economics, often succeeds in making patent nonsense of it."