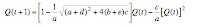1.0 IntroductionThis post duplicates an example in Richard M. Goodwin's
Chaotic Economic Dynamics (Oxford University Press, 1990). At least, I think it does, but without the typographic errors that I think are in Goodwin's book. My Figure 3 is Goodwin's Figure 2.1, and my Figure 2 is Goodwin's Figures 2.2, and 2.3.
I have no plans to prepare a Part 2 to post later. But I describe in the conclusion below why there should be a Part 2.
2.0 Supply and DemandThis model is a partial equilibrium model with well-behaved supply and demand curves. It is an internal exploration of a mainstream textbook model. The demand curve shows the price that must instantaneously prevail if the quantity on the market is to be sold:
 (1)
(1)
where
p(
t) is the price of the commodity at time
t and
q(
t) is the quantity supplied or demanded.
Time is discrete in this model, and the supply curve contains a lag. Firms plan the quantity to supply in the next period based on the price in this period:
 (2)
(2)
The supply curve shows "adaptive expectations". Economists such as Lucas have criticized the assumption of adaptive expectations. I think that critique may be inapplicable in a model with the behavior illustrated in Figure 5 below.
It's easy enough to solve for equilibrium, in which the quantity supplied and the quantity demanded are equal and do not change through time. Equation 3 gives the equilibrium quantity:
 (3)
(3)
Figure 1 illustrates. The supply and demand curves are shown. The solid dot is the equilibrium. A hint at the dynamics is also shown. At time
t, the indicated quantity is thrown on the market. One reads the price at that time off the demand curve. The quantity supplied in the next period is found from drawing a horizontal line from that intersection with the demand curve to the supply curve. This point of intersection with the supply curve is the quantity supplied in the next period. Proceeding in this way, one draws a figure that resembles a cobweb. Thus, this model is known as the cobweb model.
 |
| Figure 1: Supply and Demand |
I have explained the dynamics of this model above. One can approach the story with algebra and obtain a difference equation:
 (4)
(4)
Goodwin suggests redefining quantity as the deviation from the equilibrium quantity:
 (5)
(5)
where
Q(
t) is the redefined quantity. Equation 6 gives the difference equation in terms of the time path of the redefined quantity variable:
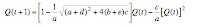 (6)
(6)
3.0 Numerical Exploration of a Special CaseGoodwin considers the special case where the parameters
b and
e are both zero. Under this special case, the difference equation becomes considerably simplified:
 (7)
(7)
Equation 7 resembles the
logistic equation. As a start at exploring the dynamics of Equation 7, consider the case where
a is unity,
c is 3/5, and
d is 21/20. (
Update: Parameters were originally specified incorrectly.) Figure 2 shows time paths for two arbitrary initial values of the redefined quantity. Both time paths converge to a two-period limit cycle. The upper extreme of the blue time path continually falls, while the upper extreme of the red path continually rises. They meet in the limit at one point in the limit cycle. The lower extremes, shown in FIgure 2, converge to the other point in the limit cycle.
 |
| Figure 2: Time Paths of Redefined Quantity |
Figure 3 shows another method for illustrating these paths. The difference equation is graphed along with a 45-degree line through the origin. One starts at an initial point along the abscissa. Drop a line to the graph of the difference equation. The value of the ordinate at this intersection with the difference equation shows the value of the redefined quantity variable at the next instant in time. Draw a horizontal line from this intersection to the 45-degree line. The value of the abscissa at this intersection to the 45-degree line is, of course, the value of the redefined quantity variable at the next instant in time. Continually in this way, one can easily trace out a time path graphically. The limit cycle is drawn in Figure 3.
 |
| Figure 3: Phase Space for These Paths |
Structural dynamics concerns how the limiting behavior varies with the parameters of a difference or differential equation. Here I only consider the effects of a fall in
a. Accordingly, Figure 4 shows the result when
a is set to 7/10, with the other parameters as in Figure 3. The limit cycle has split into a cycle of period four. This period doubling sort of bifurcation is a common approach to chaos. For some lower values of
a, the cycle will have periods eight, sixteen, thirty-two, etc. Figure 5 shows the result when
a is 2/3. I believe Figure 5 is an example of chaos, where the limit is a non-wandering set with a fractal structure. (With more exploration, I would like to see a limit cycle of period three, or some other odd value. (
Update: Try
a as 0.57.) As I understand the mathematics, period doubling can only lead to such a limit cycle with intervening chaos.)
 |
| Figure 4: Phase Space for Period Four Cycle |
 |
| Figure 5: Phase Space Showing Chaos |
4.0 ConclusionThe above exposition begins with a common introductory model in economists. And it ends with mathematical chaos. Chaos is shown in a special case in which both the demand and the supply curves go through the origin. A supply curve going through the origin, although a special case, is quite reasonable in economics. It is not economically sensible for the demand curve to go through the origin.
If there were to be a part 2 for this post, it would demonstrate the possibility of chaos in an economically relevant parameter range. One would want the demand curve to be declining throughout the first quadrant as well as intersecting the price axis at a strictly positive price. And one would want the strange attractor to lie entirely in the first quadrant for the price and untransformed quantity variables. But I haven't done enough numerical exploration yet.