On the Gain and Loss from Trade

I have written up my recent explorations in the theory of international trade.

Abstract: This article considers a model of international trade in which the number of produced commodities does not exceed the number of countries engaged in trade. Technology is modeled such that each commodity can be produced in each country from a finite series of dated labor inputs. The existence of a positive rate of profits may lead a country to specialize differently than how it would with a zero rate of profits. Trade may leave consumers in a country worse off, as compared with autarky, when the rate of profits is positive. The existence of more than two countries provides a possibility that the Production Possibilities Frontier (PPF) with trade is neither unambiguously above or below the PPF under autarky. This article re-iterates, in a setting with more than two produced commodities and more than two countries, demonstrations that the argument for free trade is logically invalid, given positive rates of profits.

Elsewhere

• Howard Reed argues we should rip up textbooks for mainstream economics and start over. (Some of what he says echoes an argument of mine.)
• I do not think Diane Coyle addresses Reed's points.
• Cahal Moran argues the problem is economics, not economists.
• Beatrice Cherrier has some frankly speculative posts on what limitations economists accepted in emphasizing tractability in developing models.
• Nathan Robinson does not like the word "neoliberalism", but understands there is a point to using it.
• David Glasner writes about Hayek's introduction of the theory of intertemporal and temporary equilibrium paths into economics. (I have no problem for those who look for Irving Fisher as a precursor of for more-or-less simultaneous discoveries in Sweden.)

Class Struggle And Specialization In International Trade

This post continues a previous numeric example. The firms in each of three countries are assumed to know a technology for producing corn, wine, and linen. The technology is such that each commodity can be produced in each country. The technology varies among countries.

Each of these small open economies can specialize and obtain non-produced commodities through foreign trade. I confine myself to patterns of specialization in which:

• Each country produces exactly one commodity domestically.
• Each commodity is produced in one country.

Six patterns of specializations meet these criteria. Table 1 lists the commodity produced in each country for each pattern of specialization.

Table 1: Patterns of Specialization

	England	Portugal	Germany
I	Corn	Wine	Linen
II	Corn	Linen	Wine
III	Wine	Corn	Linen
IV	Wine	Linen	Corn
V	Linen	Corn	Wine
V	Linen	Wine	Corn

I have found out that, in my example, each specialization is consistent with a long period position. I take the international price of corn as numeraire. That is, p₁ = 1. Table 2 lists the prices of the other commodities, p₂ and p₃, that are traded domestically. It also lists rates of profits, r₁, r₂, and r₃, in each country. This data is sufficient to calculate the wage in each country. The wage is such that supernormal profits are not earned in the commodity produced domestically, but the cost (including profits) does not exceed revenues for produced commodities. With these wages, the costs of producing a commodity in which a country does not specialize will exceed the price. It is cheaper in each country to acquire such commodities on international markets.

Table 2: International Prices and Rates of Profits

	Price of Wine	Price of Linen	Rate of Profits
			England	Portugal	Germany
I	3/4	2/3	0%	0%	0%
II	0.83	0.7	50%	0%	20%
III	0.883	3/4	20%	60%	0%
IV	1.06	1.3	60%	50%	90%
V	1.1	5/4	40%	150%	70%
VI	4/5	0.754	0%	20%	20%

The prices shown in Table 2 are not unique. For the given set of rates of profits, international prices must fall within a certain range to obtain a long period position consistent with the pattern of specialization. And rates of profits may vary in certain ranges too. I have not figured out a good way of visualizing how the spaces of prices and rates of profits is divided up by the patterns of specialization. Maybe a region for a given pattern of specialization if, contrary to this example, restitching was possible under autarky.

The numeric example illustrates that:

• The pattern of specialization in foreign trade can be driven by technology and the distribution of income.
• Only some distributions are compatible with all countries being able to specialize in a consistent way.
• The results of such specialization may not provide a country with overall gains from trade, nevermind individual groups defined by the functional distribution of income.

I have assumed constant returns to scale, but which commodity a country specializes in producing may be of importance because of considerations of learning-by-doing. Technological progress may be easier to obtain in some commodities (e.g., manufactured industrial products) than others (e.g., products of agriculture). I suspect these observations generalize to a more comprehensive Neo-Ricardian theory of trade, such as that of Yoshinori Shiozawa.

These theoretical observations, I guess, are enough to blow up much mainstream teaching on trade. I might have even made some progress on the work of Mainwaring, Metcalfe, and Steedman. Nevertheless, this model puts much aside. I am thinking of, especially, Keynesian issues of exports, imports, and effective demand; foreign exchange rates and monetary policy; and international finance.

The Gain And Loss From Trade: More On A Numeric Example

Figure 1: The Production Possibility Frontier, With And Without Trade, In "Germany"

1.0 Introduction

I continue to blunder around in parameter space in exploring my numeric example in the previous post. In this post, I continue to adopt the same assumptions for a model of three countries engaged in international trade with three produced commodities. In particular, workers are assumed to be unable to immigrate, capitalists only invest in their own country, and produced means of production are not traded. Thus, wage rates and rates of profits may vary among countries, with no tendency to change or approach equality.

2.0 Outline of the Model

For this intellectual exercise, I make the same assumptions about technology, available in each country, but differing among them. Corn, wine, and linen can each be produced from a dated series of labor inputs. Under the restrictive assumptions illustrated by the numeric example, one can rank commodities by how labor-intensive they are. Corn is most labor-intensive, and linen is least. Wine is of an intermediate labor-intensive. The endowment of labor is also taken as given.

The rate of profits is taken as given in each country. One then wants to find a set of international prices for corn, wine, and linen such that a pattern of specialization can arise for the given data. Under a model of small, open economies, the firms in each country take prices as given. In such specialization, each country will specialize in producing at least one commodity, and each commodity will be produced in one or another country. The wage in each country will be such that no pure economic profits (also known as supernormal profits) will be earned in the production of any commodities. And costs, including charges for the prevailing rate of profits, will exceed the price of all commodities that firms in each country are unwilling to produce.

3.0 Countries Specializing in Producing One Commodity

Table 1 exhibits a set of prices, for the given rates of profits, that meets these conditions. Each country specializes in the production of one commodity. England produces linen, Portugal produces corn, and Germany produces wine. The wages in each country are as shown.

Table 1: Prices with Trade

Commodity	England	Portugal	Germany
Corn	p1 = 3240/11
Wine	p2 = 324
Linen	p3 = 4050/11
Rate of Profits	r1 = 2/5	r2 = 3/2	r3 = 7/10
Wage	w1 = 4050/1199	w2 = 162/121	w3 = 1

For this particular set of prices, each country specializes differently than they would if the rates of profits were zero in each country. And they specialize differently than they would at the prices and positive rates of profits in my previous exploration of this example.

4.0 Production Possibility Frontiers (PPFs)

In the textbook theory of comparative advantage, an unambiguous gain from trade is shown by comparing the Production Possibilities Frontier (PPF) with trade and under autarky. The claim is that the with-trade PPF is moved outward from what it would be under autarky. If the consumption basket contains any commodities that must be bought on international markets, the with-trade equilibrium is supposedly unambiguously better for the country. No commodity must be consumed in a smaller amount than under autarky, and some commodities can be produced in larger quantities. Some may be hurt by trade, perhaps because they receive profits from industries whose domestic production has been replaced by imports. But they could be compensated out of the increased consumption basket, while still leaving everybody else better off in the country under consideration.

To check the textbook argument, one would look at the PPFs in each of England, Portugal, and Germany. And the textbook story is validated for England in this numeric example, with these prices and pattern of specialization. The PPF for England is rotated outwards, as compared with autarky. They coincide at the intersection with the linen axis. For every other consumption basket with non-negative quantities, the English with-trade PPF lies outside the autarkic PPF. England gains from trade.

The with-trade and autarkic PPFs for Portugal (Figure 2) replicate my previous finding that specialization can result in a loss from trade. The argument from comparative advantage is logically invalid, given positive rates of profits among countries engaged in international trade. The with-trade PPF in Portugal is rotated inwards. Portugal is unambiguously worse off with trade.

Figure 2: The Production Possibility Frontier, With And Without Trade, In "Portugal"

The story from Germany illustrates a possibility that cannot arise in the two-country, two-commodity model. The with-trade PPF (Figure 1) is neither rotated outwards nor inwards, as compared with the autarkic PPF. Along one dimension (linen), the with-trade PPF lies outside the autarkic PPF. Along another dimension (corn), it lies inside the autarkic PPF. Whether Germany is worse or better off with-trade depends on the composition of the commodity basket.

5.0 Remarks on Krugman and Obstfeld

I am unsure what I think of Krugman and Obstfeld, so far. Chapter 2 presents the argument from comparative advantage. They hammer home that, in the Ricardian model, countries are better off with-trade, no question about it. In this chapter, inputs consist only of labor, and no profits are earned. I do not know that they are clear that labor inputs are only direct. (In my example, I have labor inputs distributed over time, thereby providing a role for a rate of profits.)

In Chapter 3, they have manufacturing goods produced from labor and capital, as specified by a production function. I am not sure they ever take the marginal product of capital. They show output as a function of labor, with a diminishing marginal product. Although they do have some remarks on the supply of labor, they seem to be considering a medium term model where manufacturing output is produced with given technical conditions and a given set of production facilities. The point is to show that trade has impacts on the distribution of income in a country and can hurt some, if they are not compensated out of the supposed gains.

Chapter 4 sets out the Heckscher-Ohlin-Samuelson model in the two-factor, two-country, two-commodity case. The factors are labeled labor and land. This is as far as I've gotten in my reading.

One reading of the above is that Krugman and Obstfeld are carefully working around the Cambridge Capital Controversy. I do not know that they entirely succeed in Chapter 3. Their chapters have points, and I, of course, question their Chapter 2 claim that the gains of trade are unambiguously positive. Presumably, they would point to later chapters that put forth qualifications about imperfect competition and increasing returns to scale - a textbook presentation of the work that won Krugman a Nobel prize. Or they might point to a need in pedagogy to drum home simple points. Furthermore, their textbook, they could argues, teaches what (mainstream) economists have settled on as a consensus of what international economics is.

But what happens when the consensus has been shown to be simply wrong almost half a century ago? I know I have previously thought Krugman's offhand remarks on his blog about the CCC did not seem particularly informed. Has he ever referenced, say, Ian Steedman, in his academic work?

References

• Paul R. Krugman and Maurice Obstfeld (2003). International Economics: Theory and Policy, sixth edition.

The Loss From Trade: A Numeric Example With Three Countries And Three Produced Commodities

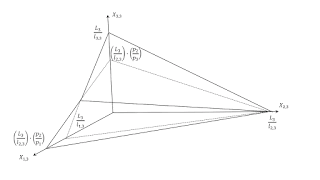
Figure 1: The Production Possibility Frontier, With And Without Trade, In "Germany"

1.0 Introduction

This post presents a numeric example of a Ricardian model of small, open economies engaged in trade. Each of three countries specializes in producing one of three commodities. Technology is modeled following an Austrian approach. Each commodity can be produced in each country from inputs of labor and "capital". Endowments of labor are taken as given parameters. It makes no sense to take the endowment of capital as a given parameter.

The with-trade Production Possibilities Frontier (PPF) can be compared to the autarkic PPF in each of the three countries. And it is unambiguously rotated inwards in one country for the set of international prices and rates of profits I consider. One cannot correctly conclude, in the traditional textbook approach, that free trade makes the inhabitants of a country better off in the aggregate.

I have previously written up an analogous argument in the two-commodity, two-country case. I should have cited Steedman and Metcalfe (1973). If I had, I would have known that my originality was less than I suggested. Shiozawa argues convincingly that the sort of model illustrated in this post can only be offered as an intellectual exercise. A more empirically applicable model would have trade in intermediate products, and the number of traded commodities would exceed the number of countries. On the other hand, I do not know of any comparable write-up of an example with three or more commodities and countries, as here.

2.0 Assumptions

Consider a model of three countries - England, Portugal, and Germany - in which three commodities are potentially traded on international markets by each country.

• Each country can produce any of the three commodities.
• The managers of firms in each country know a given flow input-point output technology with the structure described in Section 3. The technology differs among countries.
• Each country has a given endowment of labor, the only non-produced factor of production in each country. The endowment of labor may vary between countries.
• Only commodities produced for consumption can be traded internationally. Workers can neither immigrate nor emigrate. Intermediate goods, also known as capital goods, cannot be traded internationally.
• Financial capital is only invested domestically. Consequently, the rate of profits may vary across countries.
• Free competition obtains in all domestic markets; transport costs are negligible; and free trade exists in all commodities produced for consumption.

3.0 Technology

In each country, each commodity can be produced by a uniform application of labor for a specified number of years (Table 1). In each country, less total labor is required to produce a unit wine than is required to produce a unit of corn, with the labor uniformly distributed over a longer period of time in producing wine. Wine is a less labor-intensive and more capital-intensive commodity than corn, in some sense. In the same sense, linen is a less labor-intensive and more capital-intensive commodity, as compared with wine. England has an absolute advantage over Portugal, and Portugal has an absolute advantage over Germany, in producing each commodity. Nevertheless, a set of prices on international markets can exist, for corn, wine, and linen, such that firms in each country will want to specialize in producing a single commodity.

Table 1: Example Technology

Produced Commodity	Years of Labor	Labor Input per Year (Person-Yrs)
		England	Portugal	Germany
Corn	1	100	220	320
Wine	2	40	75	120
Linen	3	25	45	200/3 ≈ 66.6

The data on technology, along with the endowment of labor in each country, is enough to draw the Production Possibility Frontier (PPF) for each country in an autarky (without trade). Let X_{1, n}, X_{2, n}, and X_{3, n} be the quantity of corn, wine, and linen consumed in the nth country (n = 1, 2, 3). The plane outlined by the heavy lines in Figure 1, above, is the autarkic PPF for Germany. L₃ is the endowment of labor in Germany. l_{1, 3}, l_{2, 3}, and l_{3, 3} is the labor embodied in each commodity, when produced in Germany. These quantities are 320, 240, and 200 labor-years for this technology.

4.0 Prices and Specialization With Trade at a Rate of Profits of Zero

Next, consider an equilibrium with trade. Suppose the prices of a unit of corn, wine, and linen are as in Table 2. The question arises of whether there is a pattern of specialization among countries and a distribution of income in each country consistent with the given international prices. At this point, I take the rate of profits as zero. And I have calculated the wages shown for each country.

Table 2: Prices with Trade with Zero Rate of Profits

Commodity	England	Portugal	Germany
Corn	p1 = $300
Wine	p2 = $225
Linen	p3 = $200
Rate of Profits	r1 = 0	r2 = 0	r3 = 0
Wage	w1 = $3	w2 = $3/2	w3 = $1

Table 3 shows relative prices in each country, where the numeraire varies among countries. That is, a person-year of domestic labor is taken as the numeraire. (In Table 2, I have implicitly taken German labor - the lowest paid, as numeraire.) Notice that, in Table 3, the prices of corn in England, wine in Portugal, and linen in Germany are all equal to the labor values in the respective countries. And the prices of all other commodities falls below their labor values. Thus, since the rate of profits is zero, English firms will specialize in producing corn, Portuguese firms will produce only wine, and German firms will produce only linen. To obtain a domestic consumption basket, in any country, that contains all three commodities, each country must engage in international trade. It turns out that the PPF is unambiguously rotated outward for each country, for a pattern of specialization with a rate of profits of zero.

Table 3: Renormalized Prices with Zero Rate of Profits

Commodity	England	Portugal	Germany
Corn	p1/w1 = 100	p1/w2 = 200	p1/w3 = 300
Wine	p2/w1 = 75	p2/w2 = 150	p2/w3 = 225
Linen	p3/w1 = 200/3	p3/w2 = 400/3	p3/w3 = 200

5.0 Prices and Specialization With Trade at Positive Rates of Profits

It is well-known that, in general, prices deviate from labor values when the rate of profits is positive. Suppose the prices of corn, wine, and linen are as shown in Table 4. I also take the rates of profits as given in each country. These data yield the wages shown in the Table.

Table 4: Prices with Trade

Commodity	England	Portugal	Germany
Corn	p1 = 4275/26
Wine	p2 = 9063/52
Linen	p3 = 855/4
Rate of Profits	r1 = 3/5	r2 = 1/2	r3 = 9/10
Wage	w1 = 9063/5408	w2 = 1	w3 = 855/1664

I suppose the fractions are somewhat less awkward when normalized, as in Table 3. Unlike the case with a zero rate of profits, one should not compare these prices with labor values, in order to figure out the pattern of specialization. Rather, one should compare these prices with dated labor inputs costed up at the going rate of profits. For example, consider England. Since corn is produced with only one year of labor, its labor cost is still 100 person-years. (I am assuming wages are paid at the end of the year, not advanced.) Since p₁/w₁ is less than 100, England will import corn and not produce any. The cost of wine is 40(1 + 3/5) + 40 = 104. England will produce wine, and no super-normal profits are earned in its production. Linen is costed up as 25(1 + 3/5)² +25(1 + 3/5) + 25 = 129. p₃/w₁ is, approximately, 127.55. It is more costly to produce linen domestically, and, so, England will not do that. As a result of similar calculations, one can see that Portugal will specialize in producing linen and Germany in corn. The prices permit a consistent pattern of specialization, with all commodities being produced in some country and no firm earning more than the going rate of profits. And every country specializes in producing a different commodity shown above for the pattern with a zero rate of profits.

Table 3: Renormalized Prices

Commodity	England	Portugal	Germany
Corn	p1/w1 = 5200/53	p1/w2 = 4275/26	p1/w3 = 320
Wine	p2/w1 = 104	p2/w2 = 9063/52	p2/w3 = 1696/5
Linen	p3/w1 = 128440/1007	p3/w2 = 855/4	p3/w3 = 416

One can draw the PPFs, for each country, with this pattern of specialization and prices on international markets. The PPFs for England and Portugal are rotated out. For any consumption basket that contains some commodity not produced domestically, more is available to the country as a whole in England and Portugal. But the PPF is rotated inwards in Germany, as illustrated in Figure 1. The possibility of trade has diminished the commodities available for consumption in Germany.

6.0 Conclusion

I like that, in the above example, the pattern of specialization has each country producing a different commodity in the case with a positive rates of profits, as compared to the case with a rate of profits of zero. I'd like to convince myself that no other pattern of specialization is possible when the rate of profits is zero. I'd also like to find an example where the with-trade PPF is rotated outwards on one dimension and inwards on another. So whether every commodity in a nation's consumption basket is improved or decreased by trade would depend on its composition. I can show in the above model how a country's endowment of capital varies in value with the domestic rate of profits. And the model can be set out, in general, with any number of produced commodities and countries, with the number of commodities not exceeding the number of countries. In such a general setting, I think I will retain the severe restrictions of an Austrian model so as to exhibit that my point does not depend on, for example, capital-reversing.

It has been known for decades that the argument from comparative advantage is not a valid justification for a lack of tariffs (also known as free trade). Even setting aside such matters as, for example, increasing returns to scale or Keynesian failures of aggregate demand preventing a country from being on its PPF, the argument fails on its own terrain. This post is one more demonstration. Of course, this does not imply that any random, ill-natured, and ill-considered imposition of tariffs is likely to be a good idea in any specific case.

References

• Kurose, Kazuhiro and Naoki Yoshihara (2016). The Heckscher-Ohlin-Samuelson Model and the Cambridge Capital Controversies. Working paper.
• Metcalfe, J. S. and Ian Steedman. 1974. A Note on the Gain from Trade, Economic Record. Reprinted in Fundamental Issues in Trade Theory (Ed. by I. Steedman). Aldershot: Greg Revivals (1979, 1991).
• Shiozawa, Yoshinori. 2018. An Origin of the Neoclassical Revolution: Mill’s ‘Reversion’ and its Consequences.
• Steedman, Ian and J. S. Metcalfe. 1973. ’On Foreign Trade,’ Economia Internazionale. Reprinted in Fundamental Issues in Trade Theory (Ed. by I. Steedman). Aldershot: Greg Revivals (1979, 1991).
• Vienneau, Robert (2014). On the Loss from Trade

Trollope Trolls The Way We Live Now

A couple of months ago, I read Anthony Trollope's novel, The Way We Live Now. Even though the novel was written and set in England in 1870, I consider this post to be about contemporary American politics.

Are any of the characters in the novel sympathetic? Maybe Lady Carbury, to a certain extent, and her cousin Roger Carbury. But I want to focus on Augustus Melmotte.

Melmotte is successful in business, but is initially considered vulgar by elite socialites in London. His business success seems to be mostly a matter of a succession of cons, with a lot of juggling of accounts and debts, which he tries to avoid paying. For example, in the past he has driven a company into bankruptcy with he himself ending up with the bulk of what the stockholders had invested. (Unlike a contemporary analogue, he starts out without an inherited fortune; Melmotte is self-made.)

Melmotte decides that even though he knows nothing about what politicians are arguing about, he should run for office. In his case, he campaigns to become a Member of Parliament. Somewhat arbitrarily, he decides he is a Tory.

But there was still much to be done in London before the Goodwood week should come round in all of which Mr. Melmotte was concerned, and of much of which Mr. Melmotte was the very centre. A member for Westminster had succeeded to a peerage, and thus a seat was vacated. It was considered to be indispensable to the country that Mr. Melmotte should go into Parliament, and what constituency could such a man as Melmotte so fitly represent as one combining as Westminster does all the essences of the metropolis? There was the popular element, the fashionable element, the legislative element, the legal element, and the commercial element. Melmotte undoubtedly was the man for Westminster. His thorough popularity was evinced by testimony which perhaps was never before given in favour of any candidate for any county or borough. In Westminster there must of course be a contest. A seat for Westminster is a thing not to be abandoned by either political party without a struggle. But, at the beginning of the affair, when each party had to seek the most suitable candidate which the country could supply, each party put its hand upon Melmotte. And when the seat, and the battle for the seat, were suggested to Melmotte, then for the first time was that great man forced to descend from the altitudes on which his mind generally dwelt, and to decide whether he would enter Parliament as a Conservative or a Liberal. He was not long in convincing himself that the Conservative element in British Society stood the most in need of that fiscal assistance which it would be in his province to give; and on the next day every hoarding in London declared to the world that Melmotte was the Conservative candidate for Westminster. It is needless to say that his committee was made up of peers, bankers, and publicans, with all that absence of class prejudice for which the party has become famous since the ballot was introduced among us. Some unfortunate Liberal was to be made to run against him, for the sake of the party; but the odds were ten to one on Melmotte.

Melmotte can find some to recognize his qualifications.

The new farthing newspaper, "The Mob," was already putting Melmotte forward as a political hero, preaching with reference to his commercial transactions the grand doctrine that magnitude in affairs is a valid defense for certain irregularities.

He cannot campaign on issues, since he knows nothing about them.

There was one man who thoroughly believed that the thing at the present moment most essentially necessary to England's glory was the return of Mr. Melmotte for Westminster. This man was undoubtedly a very ignorant man. He knew nothing of any one political question which had vexed England for the last half century,—nothing whatever of the political history which had made England what it was at the beginning of that half century. Of such names as Hampden, Somers, and Pitt he had hardly ever heard. He had probably never read a book in his life. He knew nothing of the working of parliament, nothing of nationality,—had no preference whatever for one form of government over another, never having given his mind a moment's trouble on the subject. He had not even reflected how a despotic monarch or a federal republic might affect himself, and possibly did not comprehend the meaning of those terms. But yet he was fully confident that England did demand and ought to demand that Mr. Melmotte should be returned for Westminster. This man was Mr. Melmotte himself.

His campaign is mostly petty personalities. The discourse in the press is just as elevated:

Now the "Evening Pulpit," in its endeavor to make the facts of this transaction known, had placed what it called the domicile of this company in Paris, whereas it was ascertained that its official head-quarters had in truth been placed at Vienna. Was not such a blunder as this sufficient to show that no merchant of higher honor than Mr. Melmotte had ever adorned the Exchanges of modern capitals? And then two different newspapers of the time, both of them antagonistic to Melmotte, failed to be in accord on a material point. One declared that Mr. Melmotte was not in truth possessed of any wealth. The other said that he had derived his wealth from those unfortunate shareholders. Could anything betray so bad a cause as contradictions such as these? Could anything be so false, so weak, so malignant, so useless, so wicked, so self-condemned, - in fact, so "Liberal" as a course of action such as this? The belief naturally to be deduced from such statements, nay, the unavoidable conviction on the minds - of, at any rate, the Conservative newspapers - was that Mr. Melmotte had accumulated an immense fortune, and that he had never robbed any shareholder of a shilling.

He continues in his ignorance after he gets elected. He neither understands nor wants to know the conventions governing parliamentary debate. He does not address the chair, nor refer to his fellow members as "the honorary member from" wherever. Instead, all he can do is blurt out, "He's wrong", in dealing with the previous speaker. He stands up because of some petty dislike. But Trollope says that, in this case, Melmotte actually knows something about the topic, it being foreign exchange rates. But Melmotte is almost completely inarticulate.

He continues his business. He had hoped that his political eminence would contribute towards his financial interests. On the contrary, it leads to exposure of his shenanigans:

How would things go with him? What would be the end if it? - Ruin; - yes but there were worse things than ruins. And a short time since he had been so fortunate; - had made himself so safe! As he looked back at it, he could hardly say how it had come to pass that he had laid down for himself. He had known that ruin would come, and had made himself so comfortably safe, so brilliantly safe, in spite of ruin. But insane ambition had driven himself away from his anchorage. He told himself over and over again that the fault had been not in circumstances - not in that which men call Fortune, - but in his own incapacity to bear his position. He saw it now. He felt it now. If he could only begin again, how different would his conduct be!